• Welcome to the Kancolle Wiki!
  • If you have any questions regarding site content, account registration, etc., please visit the KanColle Wiki Discord

Difference between revisions of "User:Remi Scarlet/Blog/FurutakaKaiNiCosplay/Day6"

From Kancolle Wiki
Jump to navigation Jump to search
 
(2 intermediate revisions by the same user not shown)
Line 1: Line 1:
−
=='''Day 6'' '''''New!'''''==
+
==Day 6==
 
Date: 5/28/15(A tiny bit on 5/27/15 as well)<br />
 
Date: 5/28/15(A tiny bit on 5/27/15 as well)<br />
 
Time spent: ~4 hours
 
Time spent: ~4 hours
  
−
<span class="mw-customtoggle-furutaka-day6" style="border:1px solid blue; border-radius: 5px;padding:5px">Show/Hide Day 5</span>
+
<span class="mw-customtoggle-furutaka-day6" style="border:1px solid blue; border-radius: 5px;padding:5px">Show/Hide Day 6</span>
 
<div class="mw-collapsible mw-collapsed" id="mw-customcollapsible-furutaka-day6">
 
<div class="mw-collapsible mw-collapsed" id="mw-customcollapsible-furutaka-day6">
 
===5/27===
 
===5/27===
Line 41: Line 41:
 
|<math>\tau_b = m_{b}*d_{{CoM}_b} = 219.56g</math>⋅<math>in</math><br /><math>\tau_b = 219.56</math>⋅<math>in*\frac{1kg}{1000g}*\frac{2.54cm}{1in}</math><br /><math>\tau_b = 0.558kg</math>⋅<math>cm</math>
 
|<math>\tau_b = m_{b}*d_{{CoM}_b} = 219.56g</math>⋅<math>in</math><br /><math>\tau_b = 219.56</math>⋅<math>in*\frac{1kg}{1000g}*\frac{2.54cm}{1in}</math><br /><math>\tau_b = 0.558kg</math>⋅<math>cm</math>
 
|}
 
|}
−
<math>\tau_{total} = 0.127kg</math>⋅<math>cm + 0.558kg</math>⋅<math>cm = 0.785</math>kg</math>⋅<math>cm</math>
+
<math>\tau_{total} = 0.127kg</math>⋅<math>cm + 0.558kg</math>⋅<math>cm = 0.785kg</math>⋅<math>cm</math>
  
 
And since I have two of these barrels, I needed a servo motor with at least a kg⋅cm rating of <math>0.785 * 2 = 1.57kg</math>⋅<math>cm</math>. Since it's always better to err on the side of caution, I decided to just go overkill and get a 4.1kg⋅cm rated servo.
 
And since I have two of these barrels, I needed a servo motor with at least a kg⋅cm rating of <math>0.785 * 2 = 1.57kg</math>⋅<math>cm</math>. Since it's always better to err on the side of caution, I decided to just go overkill and get a 4.1kg⋅cm rated servo.

Latest revision as of 05:41, 31 May 2015

Day 6

Date: 5/28/15(A tiny bit on 5/27/15 as well)
Time spent: ~4 hours

Show/Hide Day 6

5/27

So I'll quickly go over what I did on 5/27/15 since I didn't get a whole lot done. Basically, yesterday was spent clicking around ebay and amazon buying various parts. So things I bought include

  • Knee socks
  • Thigh highs
  • Cotton bodysuit
  • Penny loafer shoes
  • Pleated blue skirt
  • Two 4.1kg-cm rated servo motors

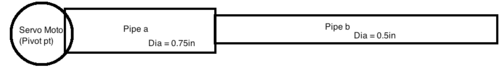
For the servo motors, they have to be able to rotate two barrels that are have two components to them of certain length. The two components have different diameters.

Furutaka cannon diagram

Where [math]\displaystyle{ a }[/math] is pipe of diameter 0.5inches and [math]\displaystyle{ b }[/math] is pipe of diameter 0.75inches.
Note that with servo motor ratings, [math]\displaystyle{ kg }[/math]⋅[math]\displaystyle{ cm }[/math], it seems that it's just it's torque without the gravity component calculated in. The [math]\displaystyle{ \tau }[/math] I use in the calculations isn't actually torque but it's the same idea so I use the symbol for it, even if it's technically incorrect.
 [math]\displaystyle{ CoM }[/math] is Center of Mass

Pipe [math]\displaystyle{ a }[/math] Pipe [math]\displaystyle{ b }[/math]
[math]\displaystyle{ \lambda_a = \frac{100g}{24inches} = 4.16\frac{g}{in} }[/math] [math]\displaystyle{ \lambda_b = \frac{150g}{24inches} = 6.24\frac{g}{in} }[/math]
Given [math]\displaystyle{ \ell_a = 7in }[/math] Given [math]\displaystyle{ \ell_b = 4in }[/math]
[math]\displaystyle{ m_a = \lambda_{a}*\ell_{a} = 24.96g }[/math] [math]\displaystyle{ m_b = \lambda_{b}*\ell_{b} = 29.12g }[/math]
[math]\displaystyle{ d_{{CoM}_a} = \frac{4in}{2} = 2in }[/math] [math]\displaystyle{ d_{{CoM}_b} = 4in + \frac{7in}{2} = 7.5in }[/math]
[math]\displaystyle{ \tau_a = m_{a}*d_{{CoM}_a} = 49.92g }[/math]⋅[math]\displaystyle{ in }[/math]
[math]\displaystyle{ \tau_a = 49.92g }[/math]⋅[math]\displaystyle{ in*\frac{1kg}{1000g}*\frac{2.54cm}{1in} }[/math]
[math]\displaystyle{ \tau_a = 0.127kg }[/math]⋅[math]\displaystyle{ cm }[/math] 		[math]\displaystyle{ \tau_b = m_{b}*d_{{CoM}_b} = 219.56g }[/math]⋅[math]\displaystyle{ in }[/math]
[math]\displaystyle{ \tau_b = 219.56 }[/math]⋅[math]\displaystyle{ in*\frac{1kg}{1000g}*\frac{2.54cm}{1in} }[/math]
[math]\displaystyle{ \tau_b = 0.558kg }[/math]⋅[math]\displaystyle{ cm }[/math]

[math]\displaystyle{ \tau_{total} = 0.127kg }[/math]⋅[math]\displaystyle{ cm + 0.558kg }[/math]⋅[math]\displaystyle{ cm = 0.785kg }[/math]⋅[math]\displaystyle{ cm }[/math]

And since I have two of these barrels, I needed a servo motor with at least a kg⋅cm rating of [math]\displaystyle{ 0.785 * 2 = 1.57kg }[/math]⋅[math]\displaystyle{ cm }[/math]. Since it's always better to err on the side of caution, I decided to just go overkill and get a 4.1kg⋅cm rated servo.

Shoutout to the Kancolle USA group because I completely forgot how torque worked. It's been a few years kek. And to think I was originally a mechanical engineering major a long time ago.

That said, let's move onto what I actually did today.

5/28

So today, I decided to actually start working on the cannons since I'd been delaying them for a few days now. That said, I didn't really plan too much out other than some basic goals for dimensions. I aimed for about a 12in x 4.5in x 7.5in frame for the body and so I decided to go ahead and run with that. Images will likely serve as better explanation so I'll do that gallery thing again with explanations for the images in the annotation. Again, feel free to look through my Imgur Album for easier viewing of images.

  • Double barrel cannons for shoulder mount on Furutaka kai ni

  • Applying pressure to wood glue to elevate contact-point with board due to screw head

  • Process of cutting foamboard with an x-acto knife

  • Preliminary cutout sketch with some measurements

  • Loosely attached cutouts (preliminary)

  • Attaching cannon base to PVC frame

  • Cutout of front cross-sectional support of cannon

  • Attaching smaller pieces together before attaching to main cannon body

  • Shot of the used wood glue

  • Front wall of cannon. Note the depressed cut to allow cannon barrels to move

  • Glued and combined shot of cannon without wall coverings

  • Another shot of cannons before wall covering

  • Closer shot of cannon barrels in the frame

  • Back view of cannon

Retrieved from "https://en.kancollewiki.net/w/index.php?title=User:Remi_Scarlet/Blog/FurutakaKaiNiCosplay/Day6&oldid=61064"